Unraveling the Essence of Planar Maps: A Comprehensive Exploration
Related Articles: Unraveling the Essence of Planar Maps: A Comprehensive Exploration
Introduction
With enthusiasm, let’s navigate through the intriguing topic related to Unraveling the Essence of Planar Maps: A Comprehensive Exploration. Let’s weave interesting information and offer fresh perspectives to the readers.
Table of Content
Unraveling the Essence of Planar Maps: A Comprehensive Exploration

In the realm of mathematics, particularly within the branches of graph theory and topology, the concept of a planar map emerges as a powerful tool for visualizing and analyzing complex relationships. This article delves into the intricacies of planar maps, elucidating their fundamental definition, properties, applications, and significance.
Defining the Planar Map:
A planar map, in its simplest form, is a representation of a graph where all vertices and edges are arranged in a way that no edges cross each other. This arrangement allows for a clear and unambiguous visualization of the connections between elements, making it particularly useful for understanding networks, relationships, and spatial arrangements.
Understanding the Components:
To fully grasp the concept of a planar map, it is essential to define its constituent elements:
- Graph: A graph is a collection of vertices (nodes) and edges that connect these vertices. Vertices represent entities, and edges signify relationships between them.
- Planar Embedding: A planar embedding of a graph is a specific arrangement of vertices and edges on a plane such that no edges intersect except at shared vertices.
- Faces: In a planar map, a face refers to a region bounded by edges. This includes the unbounded region surrounding the entire map.
Properties of Planar Maps:
Planar maps possess several key properties that contribute to their significance:
- Euler’s Formula: This fundamental formula establishes a relationship between the number of vertices (V), edges (E), and faces (F) in a connected planar map: V – E + F = 2. This formula holds true for all planar maps and plays a crucial role in various graph-theoretic proofs.
- Planarity Testing: Determining whether a given graph can be represented as a planar map is a computationally significant problem. Algorithms exist to test planarity efficiently, allowing for the identification of graphs suitable for planar map representations.
- Dual Graphs: Every planar map has a corresponding dual graph, where each face in the original map corresponds to a vertex in the dual graph, and vice versa. Edges connect adjacent faces in the original map. This duality provides a powerful tool for analyzing properties of planar maps.
Applications of Planar Maps:
The versatility of planar maps extends to numerous fields, including:
- Network Analysis: Planar maps are instrumental in representing and analyzing networks, such as transportation systems, communication networks, and social networks. The visualization of connections allows for the identification of bottlenecks, optimal routes, and network resilience.
- Cartography: Planar maps are the foundation of cartography, where they are used to represent geographical features, such as roads, rivers, and cities, on a flat surface. The ability to preserve spatial relationships is crucial for accurate map creation.
- Circuit Design: In electrical engineering, planar maps are employed in the design of printed circuit boards (PCBs), where components are connected through traces on a planar surface. Planar maps facilitate the optimization of circuit layout, minimizing wire crossings and improving performance.
- Computational Geometry: Planar maps find applications in computational geometry problems, such as triangulation, polygon intersection, and point location. Their ability to represent spatial relationships efficiently makes them valuable tools for geometric computations.
- Graph Drawing: Planar maps are crucial for graph drawing algorithms, which aim to produce aesthetically pleasing and informative representations of graphs. Planarity ensures the avoidance of edge crossings, improving readability and understanding.
Benefits of Utilizing Planar Maps:
The use of planar maps offers several advantages:
- Clarity and Visualization: The absence of edge crossings in planar maps enhances clarity and facilitates easy visualization of connections and relationships. This is particularly beneficial for complex networks and spatial arrangements.
- Efficient Analysis: Planar maps lend themselves to efficient analysis, as algorithms can be developed that exploit the inherent structure of the map. This leads to faster computations and more accurate results.
- Reduced Complexity: By eliminating edge crossings, planar maps simplify the representation of graphs, reducing the overall complexity and facilitating easier comprehension.
- Intuitive Understanding: The visual representation of planar maps provides an intuitive understanding of the relationships between entities, making it easier for individuals to grasp complex concepts.
FAQs About Planar Maps:
Q: What is the difference between a planar graph and a planar map?
A: A planar graph is a graph that can be embedded in a plane without edge crossings. A planar map is a specific embedding of a planar graph, including the arrangement of vertices and edges on the plane and the resulting faces.
Q: Can all graphs be represented as planar maps?
A: No. Only graphs that are planar can be represented as planar maps. There are graphs, such as the complete graph K5 and the complete bipartite graph K3,3, that are non-planar and cannot be embedded in a plane without edge crossings.
Q: How do I determine if a graph is planar?
A: Several algorithms exist for testing planarity, including Kuratowski’s theorem and the Hopcroft-Tarjan algorithm. These algorithms efficiently determine whether a graph can be embedded in a plane without edge crossings.
Q: What are some real-world examples of planar maps?
A: Road maps, subway maps, circuit diagrams, and social network diagrams are all examples of planar maps. These representations use planar embeddings to visualize connections and relationships in a clear and understandable manner.
Q: How do planar maps relate to topology?
A: Planar maps are closely related to the field of topology, which deals with the study of geometric shapes and their properties. The concept of planar embedding is a fundamental concept in topology, and planar maps provide a visual representation of topological concepts.
Tips for Working with Planar Maps:
- Choose the Right Representation: Consider the nature of the data and the relationships being visualized when selecting a planar map representation. Different types of planar maps, such as orthogonal or radial layouts, can be more suitable for specific purposes.
- Optimize for Clarity: Aim to create planar maps that are easy to understand and interpret. Avoid unnecessary clutter and use clear labeling and visual cues to enhance readability.
- Leverage Duality: Utilize the concept of dual graphs to gain insights into the properties of planar maps. The dual graph can provide valuable information about connectivity, flow, and other aspects of the original map.
- Employ Planarity Testing Algorithms: When dealing with large or complex graphs, use planarity testing algorithms to determine if a graph can be represented as a planar map. This can help avoid unnecessary attempts at embedding non-planar graphs.
Conclusion:
Planar maps serve as a powerful tool for visualizing and analyzing complex relationships in various fields. Their ability to represent graphs without edge crossings enhances clarity, facilitates efficient analysis, and promotes intuitive understanding. From network analysis and cartography to circuit design and computational geometry, planar maps find widespread applications, contributing significantly to our understanding of interconnected systems and spatial arrangements. As we continue to explore the intricacies of graphs and networks, planar maps remain an indispensable tool for unraveling the complex relationships that shape our world.

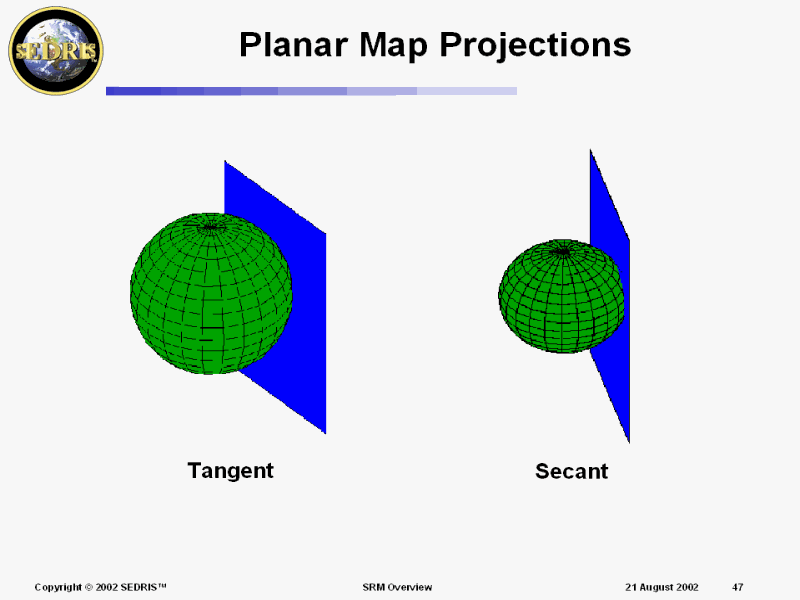

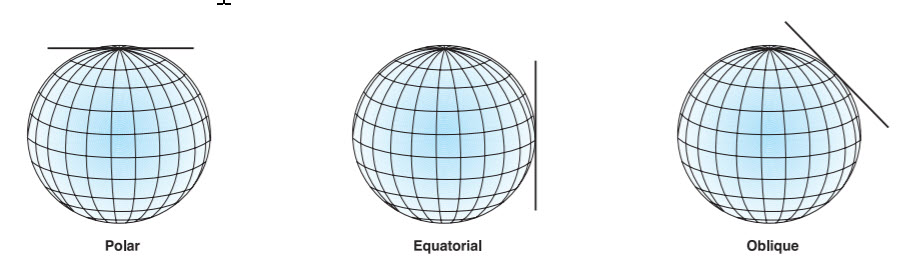
![[PDF] Planar Maps as Labeled Mobiles Semantic Scholar](https://d3i71xaburhd42.cloudfront.net/966e370c214949110549dda979107ac38017ec01/21-Figure13-1.png)

Closure
Thus, we hope this article has provided valuable insights into Unraveling the Essence of Planar Maps: A Comprehensive Exploration. We hope you find this article informative and beneficial. See you in our next article!